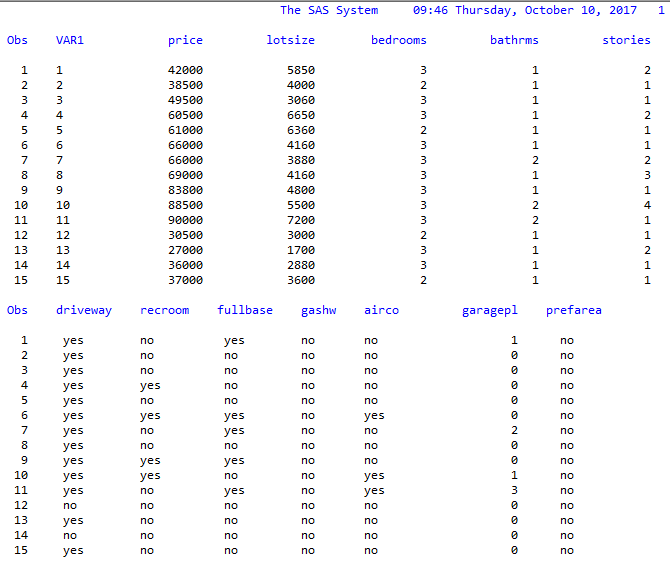
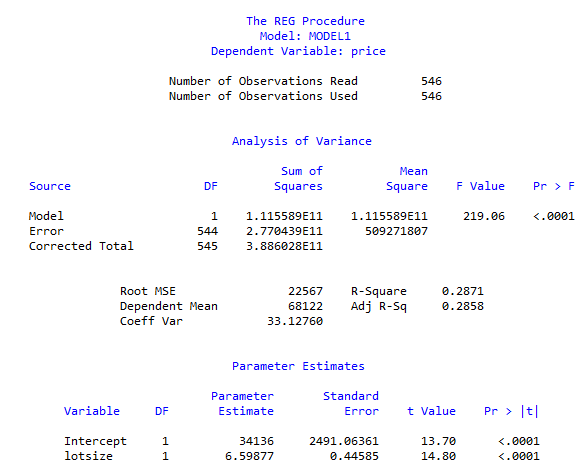
Chapter 8 Certification Chapter 8 Review Questions in Statistics
Hawkes Learning Chapter 8 Certification Chapter 8 Review Questions in Statistics
Qn1. direct mail company wishes to estimate the proportion of people on a large mailing list that will purchase a product. Suppose the true proportion is 0.07. If 402 are sampled, what is the probability that the sample proportion will be less than 0.04 ? Round your answer to four decimal places.
Qn2. Suppose a large shipment of laser printers contained 17% defectives. If a sample of size 224 is selected, what is the probability that the sample proportion will be greater than 16%? Round your answer to four decimal places.
Qn3. A carpet expert believes that 7% of Persian carpets are counterfeits. If the expert is accurate, what is the probability that the proportion of counterfeits in a sample of 574 Persian carpets would be less than 6%? Round your answer to four decimal places.
Qn4. The mean life of a television set is 138 months with a variance of 324. If a sample of 83 televisions is randomly selected, what is the probability that the sample mean would differ from the true mean by less than 5.4 months? Round your answer to four decimal places.
Qn5. A courier service company wishes to estimate the proportion of people in various states that will use its services. Suppose the true proportion is 0.07. If 330 are sampled, what is the probability that the sample proportion will differ from the population proportion by greater than 0.03? Round your answer to four decimal places.
Qn6 . If 330 are sampled, what is the probability that the sample proportion will differ from the population proportion by greater than 0.03? Round your answer to four decimal places.
Qn6. Suppose babies born in a large hospital have a mean weight of 3225 grams, and a standard deviation of 535 grams. If 106 babies are sampled at random from the hospital, what is the probability that the mean weight of the sample babies would differ from the population mean by more than 53 grams? Round your answer to four decimal places.
Qn7. Suppose 55% of the population has a college degree. If a random sample of size 496 is selected, what is the probability that the proportion of persons with a college degree will differ from the population proportion by less than 4%? Round your answer to four decimal places.
Qn8. The mean points obtained in an aptitude examination is 183 points with a standard deviation of 13 points. What is the probability that the mean of the sample would be greater than 186.2 points if 73 exams are sampled? Round your answer to four decimal places.
Qn9. Suppose cattle in a large herd have a mean weight of 1158lbs and a standard deviation of 92lbs. What is the probability that the mean weight of the sample of cows would be less than 1149lbs if 55 cows are sampled at random from the herd? Round your answer to four decimal places.
Qn10. Thompson and Thompson is a steel bolts manufacturing company. Their current steel bolts have a mean diameter of 135 millimeters, and a variance of 64. If a random sample of 32 steel bolts is selected, what is the probability that the sample mean would be less than 133 millimeters? Round your answer to four decimal places.